How To Draw Derivative Of A Graph
How To Draw Derivative Of A Graph - Draw turning points at the location of any inflection points. Trust me, it’s straightforward, and you’ll get the hang of it in no time. Plot a function and its derivative, or graph the derivative directly. Use concavity and inflection points to explain how the sign of the second derivative affects the shape of a function’s graph. Web differentiation allows us to determine the change at a given point. Describe three conditions for when a function does not have a derivative Find space on a flat surface for you to work on. State the first derivative test for critical points. State the connection between derivatives and continuity. Remember, this graph represents the derivative of a function. Find space on a flat surface for you to work on. State the connection between derivatives and continuity. Use a straightedge to draw a tangent line at the point on the graph that you want to estimate the derivative for. F x = 3x2 − 6x + 2. First, notice that the derivative is equal to 0 when x =. Log in or sign up. Exercise \ (\pageindex {1}\) exercise \ (\pageindex {2}\) exercise \ (\pageindex {3}\) exercise \ (\pageindex {4}\) stage 2. Describe three conditions for when a function does not have a derivative Y − f a = g a x − a. Remember, this graph represents the derivative of a function. 732k views 7 years ago. Web for the following exercises, draw a graph that satisfies the given specifications for the domain x ϵ [−3, 3]. G x = d dx f x −5 ≤ x ≤ a. Use concavity and inflection points to explain how the sign of the second derivative affects the shape of a function’s graph. Exercise \. G x = d dx f x −5 ≤ x ≤ a. Draw turning points at the location of any inflection points. Describe three conditions for when a function does not have a derivative. Web for the following exercises, draw a graph that satisfies the given specifications for the domain x ϵ [−3, 3]. 732k views 7 years ago. The derivative describes how the slope of a curve changes as x, the horizontal value, changes. This video contains plenty of examples and. Web derivative grapher | desmos. If you guys have any youtube videos to share as well, please send link! Explain the relationship between a function and its first and second derivatives. Sketching the derivative of a function. Sketch your graph by plotting a few points (from step 1) and connecting them with curved lines (for a polynomial function) or straight lines (for a linear function or absolute value function ). Web for the following exercises, draw a graph that satisfies the given specifications for the domain x ϵ [−3, 3]. This. Web analyzing three graphs to see which describes the derivative of which other graph. The derivative describes how the slope of a curve changes as x, the horizontal value, changes. Graph functions, plot points, visualize algebraic equations, add sliders, animate graphs, and more. State the connection between derivatives and continuity. Web thanks to all of you who support me on. Mark zeros at the locations of any turning points or stationary inflection points. G x = d dx f x −5 ≤ x ≤ a. Trust me, it’s straightforward, and you’ll get the hang of it in no time. Sketch your graph by plotting a few points (from step 1) and connecting them with curved lines (for a polynomial function). Explain the relationship between a function and its first and second derivatives. Examine an original graph that is on a coordinate plane of a function that you can differentiate (make a derivative of. State the connection between derivatives and continuity. The function does not have to be continuous or differentiable. The derivative describes how the slope of a curve changes. Find space on a flat surface for you to work on. F x = 3x2 − 6x + 2. Use a straightedge to draw a tangent line at the point on the graph that you want to estimate the derivative for. Remember, this graph represents the derivative of a function. The function does not have to be continuous or differentiable. If you guys have any youtube videos to share as well, please send link! State the connection between derivatives and continuity. Graph functions, plot points, visualize algebraic equations, add sliders, animate graphs, and more. Use concavity and inflection points to explain how the sign of the second derivative affects the shape of a function’s graph. Web derivative grapher | desmos. Sketching the derivative of a function. Log in or sign up. F x = 3x2 − 6x + 2. Here we have the graph of the derivative f' (x) = x. Draw turning points at the location of any inflection points. State the first derivative test for critical points. Mark zeros at the locations of any turning points or stationary inflection points. Trust me, it’s straightforward, and you’ll get the hang of it in no time. Use concavity and inflection points to explain how the sign of the second derivative affects the shape of a function’s graph. Exercise \ (\pageindex {10}\) stage 1. Describe three conditions for when a function does not have a derivative
How to Sketch the Graph of the Derivative
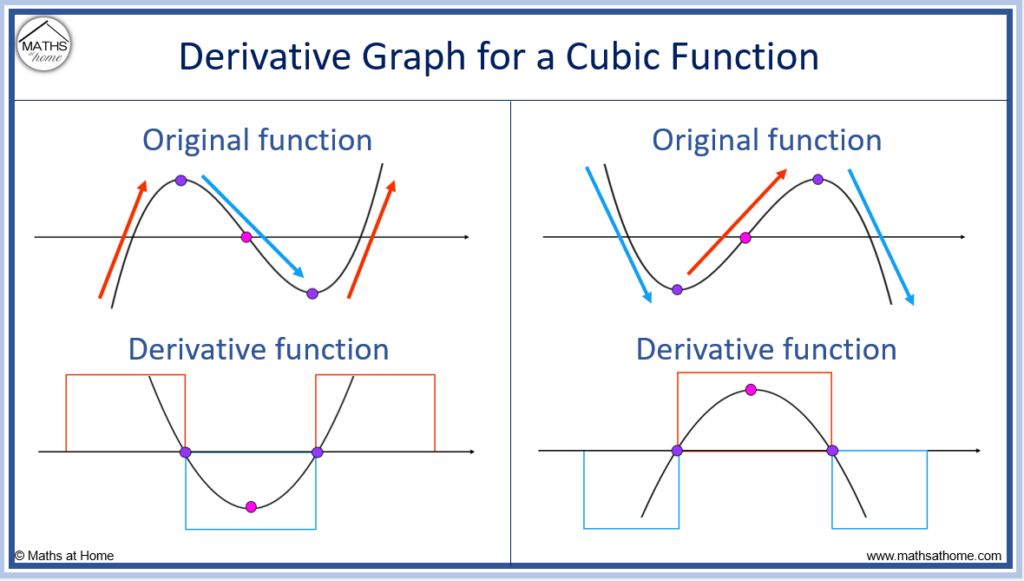
How to Sketch the Graph of the Derivative

Draw the Function given Graph of Derivative YouTube

How to sketch first derivative and Function from graph of second
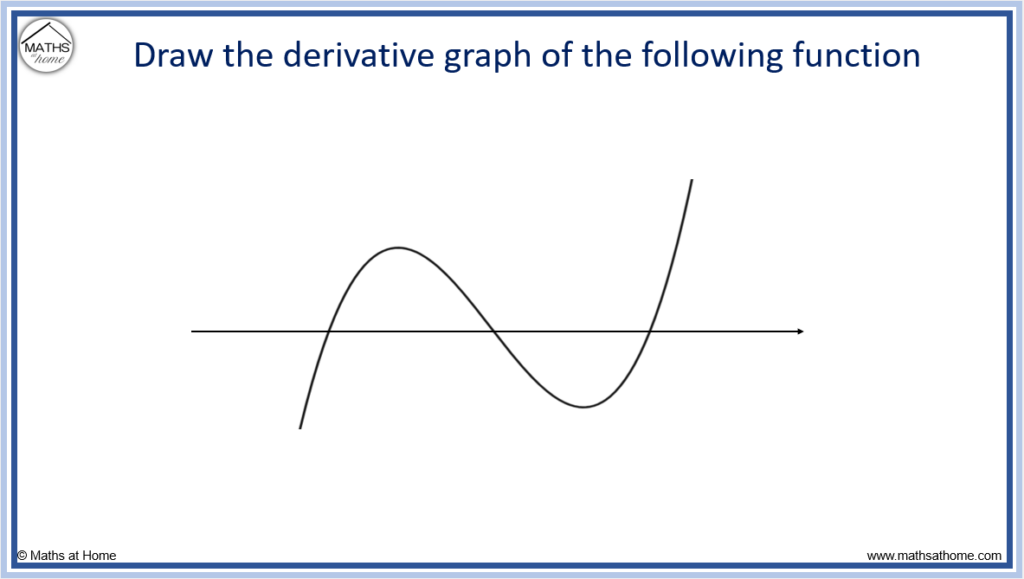
How to Sketch the Graph of the Derivative

Drawing the Graph of a Derivative YouTube
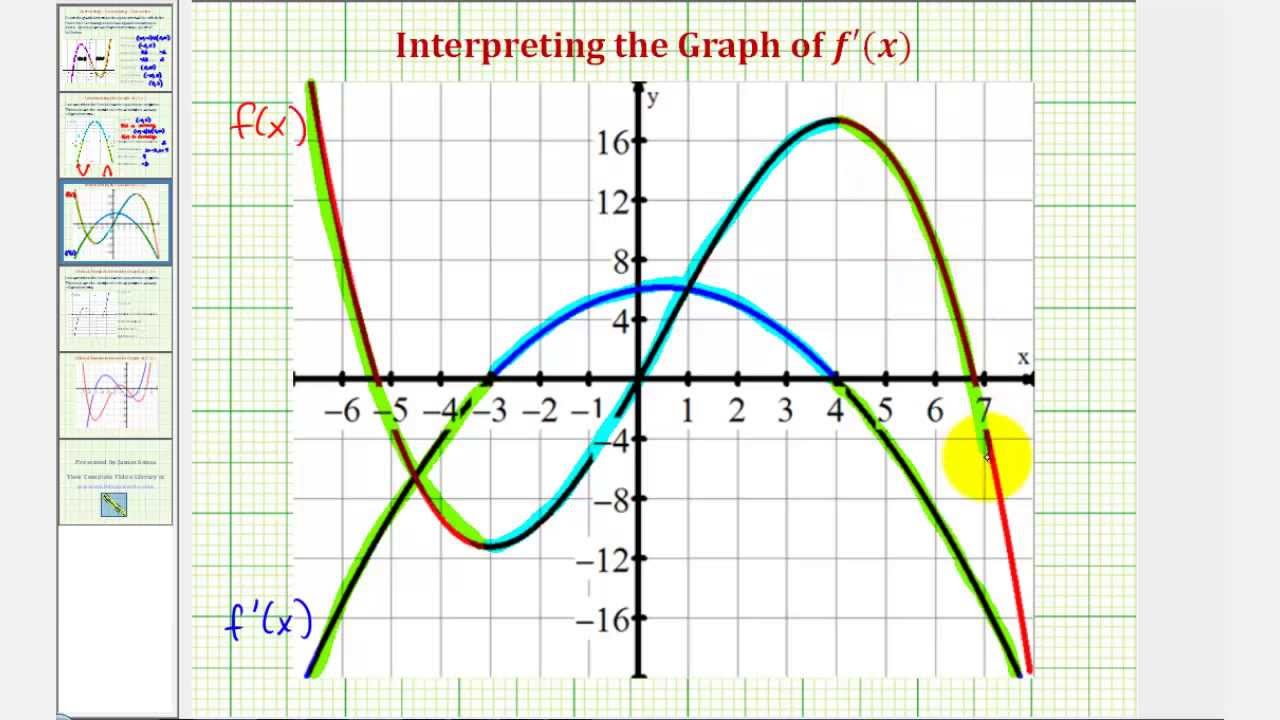
Ex 1 Interpret the Graph of the First Derivative Function Degree 2
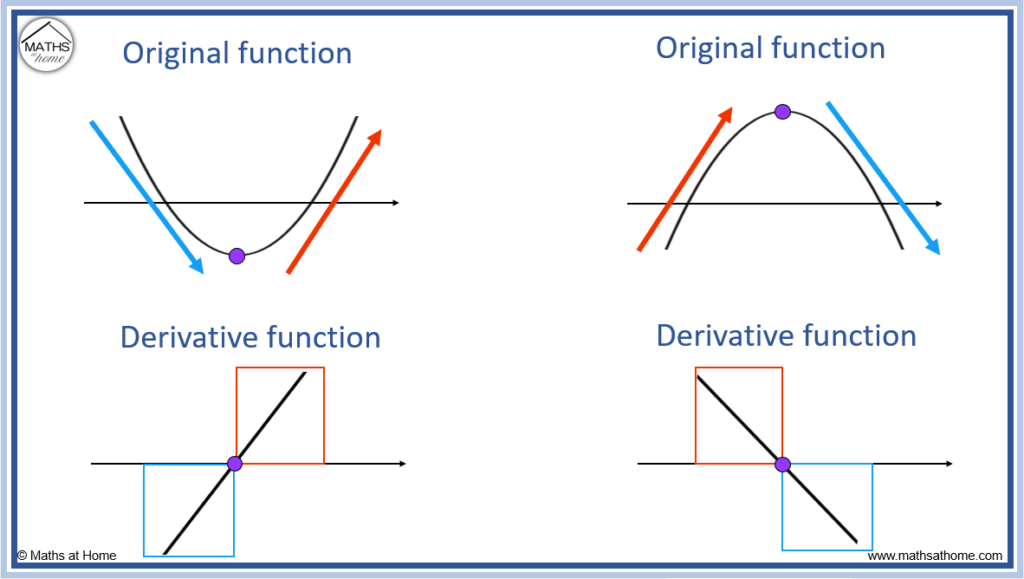
How to Sketch the Graph of the Derivative
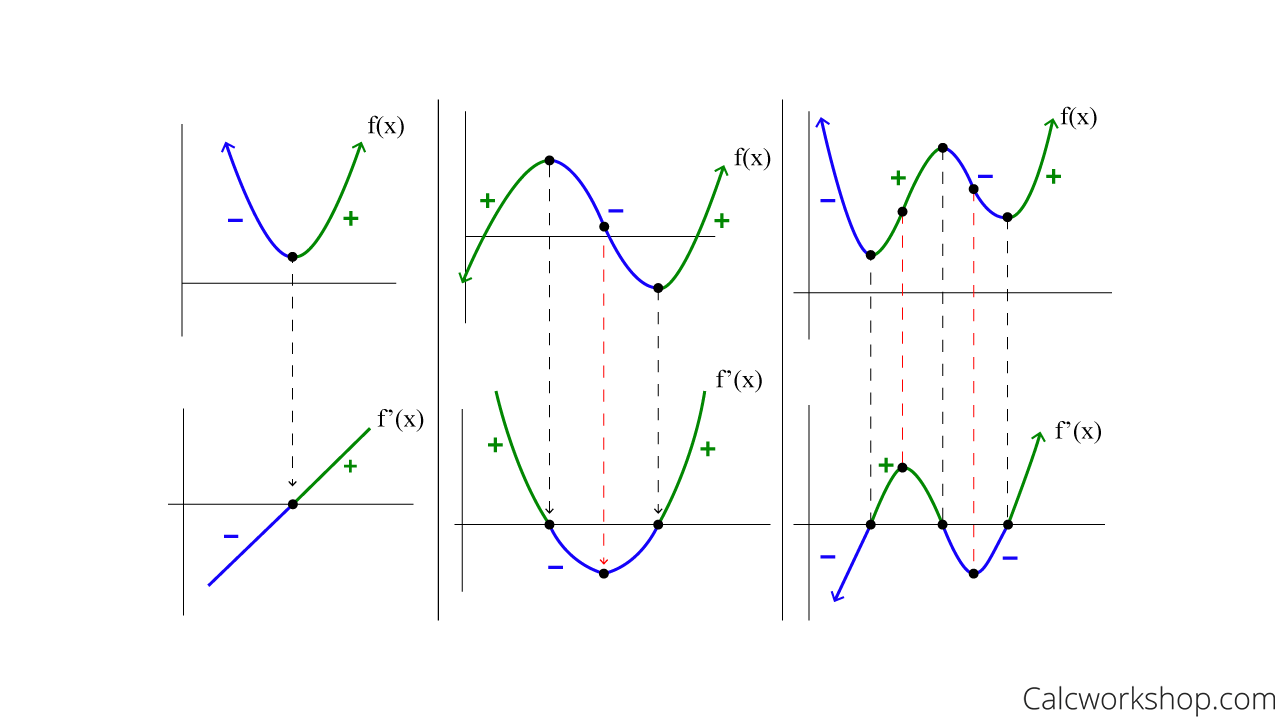
Derivative Graph Vs Original Function (w/ 15+ Examples!)
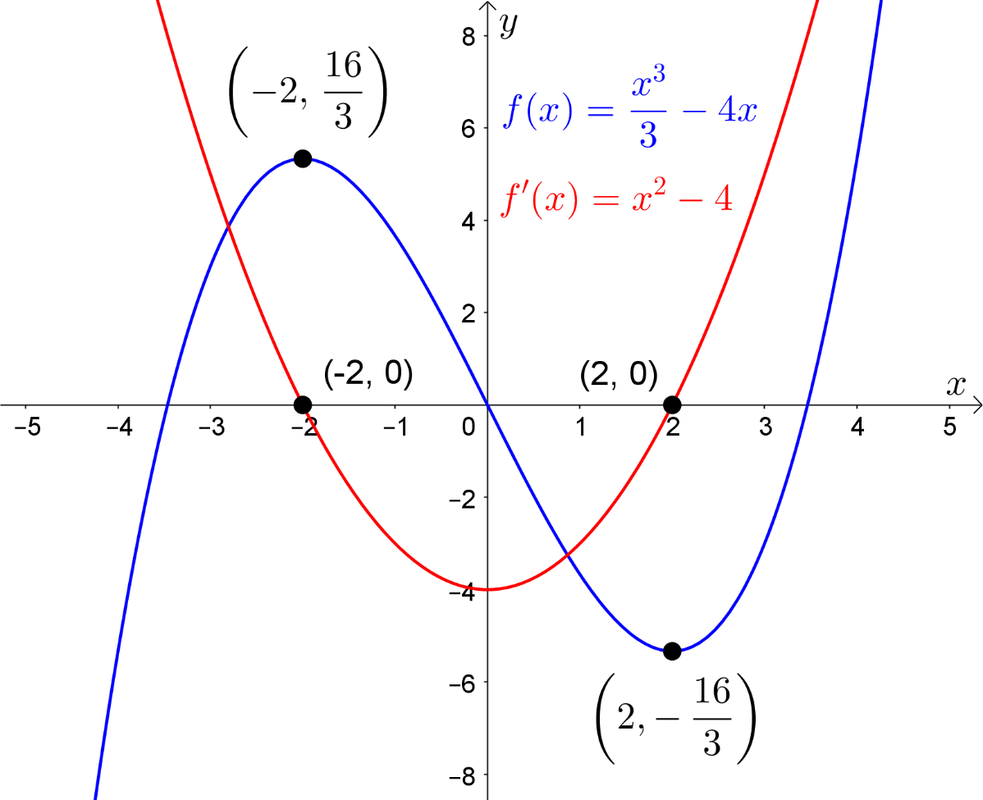
6D Graphs of derivative functions OLVER EDUCATION
Web Explore Math With Our Beautiful, Free Online Graphing Calculator.
F X = X 3 − 4.
Web I Am Having A Really Hard Time Understanding A Derivative Graph And How I Can Reference It To Draw The Original Graph.
Our Task Is To Find A Possible Graph Of The Function.
Related Post: