Derivative And Integral Chart
Derivative And Integral Chart - Web those would be derivatives, definite integrals, and antiderivatives (now also called indefinite integrals). Equation y = f ( ax + by + k ) is transformed to separable with substitution u = ax + by + k. Web 3.1 defining the derivative; 3.9 derivatives of exponential and logarithmic functions Walk slow, the distance increases slowly. Cf(x) = cf0(x), c is any constant. A distance increase of 4 km in 1 hour gives a speed of 4 km per hour. If the power n of cosine is odd (n = 2k + 1), save one cosine factor and use cos2(x) = 1 express the rest of the factors in terms of sine: Web table of derivatives and integrals. Stand still and the distance won't change. Trig substitutions if the integral contains the following root use the given substitution and formula. Let's start by looking at sums and slopes: Web draw a graph of any function and see graphs of its integral, first derivative, and second derivative. ()0 d c dx =, c is any constant. 2 + 1 ( n + 1 ) x dx. Walk slow, the distance increases slowly. (6) ∫x(x + a)ndx = (x + a)n + 1((n + 1)x − a) (n + 1)(n + 2) (7) When you learn about the fundamental theorem of calculus, you will learn that the antiderivative has a very, very important property. ∫ 1 (x + a)2dx = − 1 x + a. 3.7 derivatives. Geometrically the differentiation and integration formula is used to find the slope of a curve,. D dx(f(x) g(x)) = f′(x) g(x) + f(x)g′ (x) d d x ( f ( x) g ( x)) = f ′ ( x) g ( x) + f ( x) g ′ ( x) 4. Web differentiation is used to break down the function. (5) ∫(x + a)ndx = (x + a)n + 1 n + 1, n ≠ − 1. Web 3.1 defining the derivative; D dx(c) = 0 d d x ( c) = 0. (3) ∫ 1 ax + bdx = 1 a ln |ax + b|. (4) integrals of rational functions. When you learn about the fundamental theorem of calculus, you will learn that the antiderivative has a very, very important property. A distance increase of 4 km in 1 hour gives a speed of 4 km per hour. Let's start by looking at sums and slopes: Web table of basic integrals. (4) integrals of rational functions. ∫ 1 (x + a)2dx = − 1 x + a. D dx(f(x)g(x)) = f'(x)g(x) + f(x)g'(x) d d x ( f ( x) g ( x)) = f ′ ( x) g ( x) + f ( x) g ′ ( x) 4. Web we begin with the derivatives of the sine and cosine functions and then use them. 1, n is any number. Web table of derivatives and integrals. The integral of a function between an upper and lower limit. D dx(xn) = nxn−1, for real numbersn d d x (. ∫ 1 (x + a)2dx = − 1 x + a. D dx(f(x)g(x)) = f'(x)g(x) + f(x)g'(x) d d x ( f ( x) g ( x)) = f ′ ( x) g ( x) + f ( x) g ′ ( x) 4. Web the table below shows you how to differentiate and integrate 18 of the most common functions. D dx(f(x) g(x)) = f′(x) g(x) + f(x)g′ (x) d. Walking in a straight line. ∫ 1 (x + a)2dx = − 1 x + a. (1) ∫1 xdx = ln |x|. Let's start by looking at sums and slopes: Web table of derivatives and integrals. Is transformed to separable with substitution u = y. Sum difference rule \left (f\pm g\right)^'=f^'\pm g^' constant out \left (a\cdot f\right)^'=a\cdot f^' product rule (f\cdot g)^'=f^'\cdot g+f\cdot g^' Being able to calculate the derivatives of the sine and cosine functions will enable us to find the velocity and acceleration of simple harmonic motion. Cf(x) = cf0(x), c is any constant.. Web differentiation is used to break down the function into parts, and integration is used to unite those parts to form the original function. Web we begin with the derivatives of the sine and cosine functions and then use them to obtain formulas for the derivatives of the remaining four trigonometric functions. © 2005 paul dawkins derivatives basic properties/formulas/rules d(cf()x)cfx() dx =¢, c is any constant. Walking in a straight line. D dx(c) = 0 d d x ( c) = 0. The integral of a function between an upper and lower limit. Cf(x) = cf0(x), c is any constant. (2) ∫udv = uv − ∫vdu. ()0 d c dx =, c is any constant. D dx(f(x) + g(x)) = f′(x) + g′(x) d d x ( f ( x) + g ( x)) = f ′ ( x) + g ′ ( x) 3. Let's start by looking at sums and slopes: Web draw a graph of any function and see graphs of its integral, first derivative, and second derivative. When you learn about the fundamental theorem of calculus, you will learn that the antiderivative has a very, very important property. (3) ∫ 1 ax + bdx = 1 a ln |ax + b|. An antiderivative is a differentiable function f whose derivative is equal to f f (i.e., f'=f f ′ = f ). Being able to calculate the derivatives of the sine and cosine functions will enable us to find the velocity and acceleration of simple harmonic motion.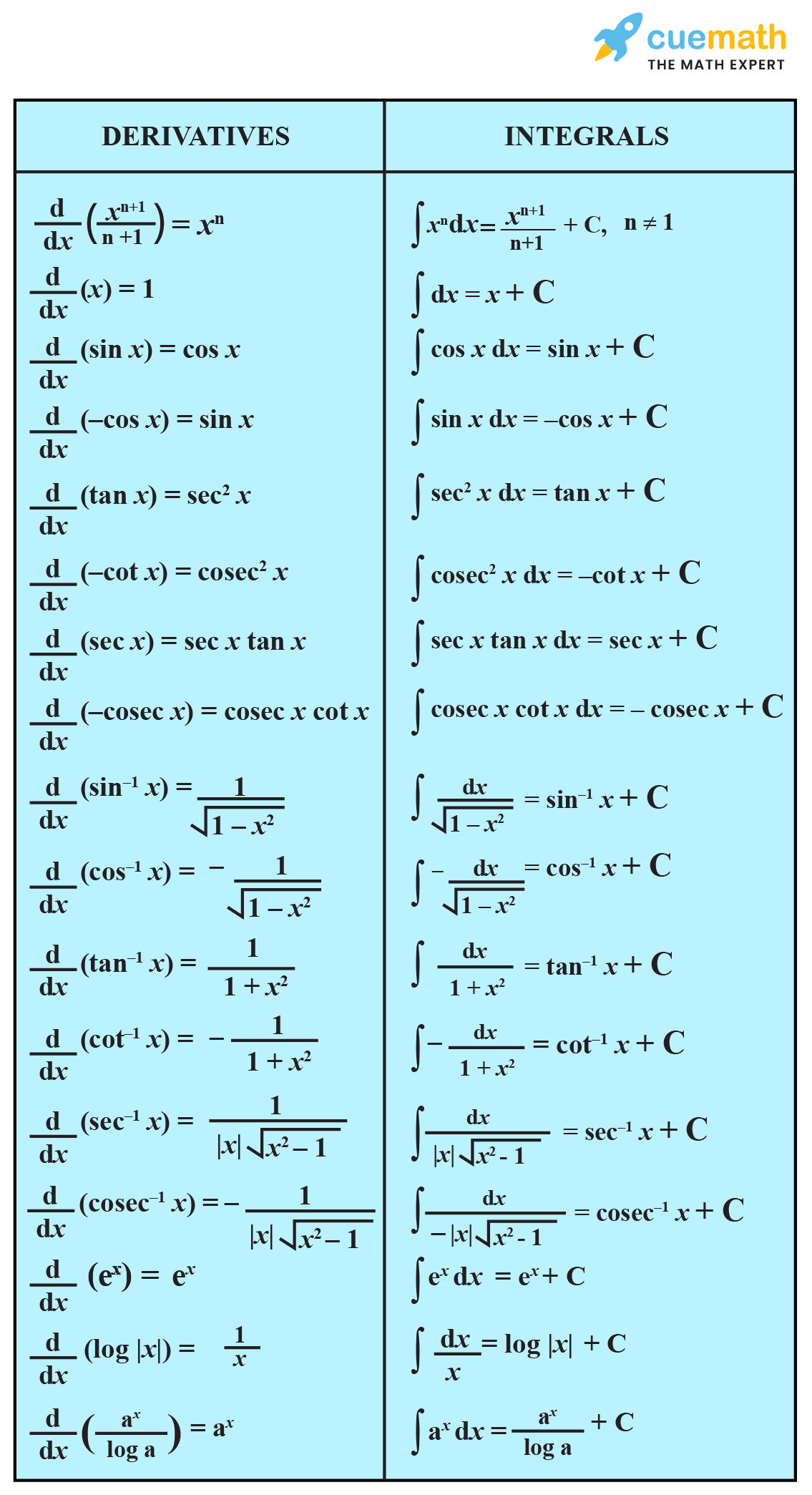
Printable Table Of Integrals

derivative and integral table Math, Derivative, Physics

Trig Integrals And Derivatives pdfshare
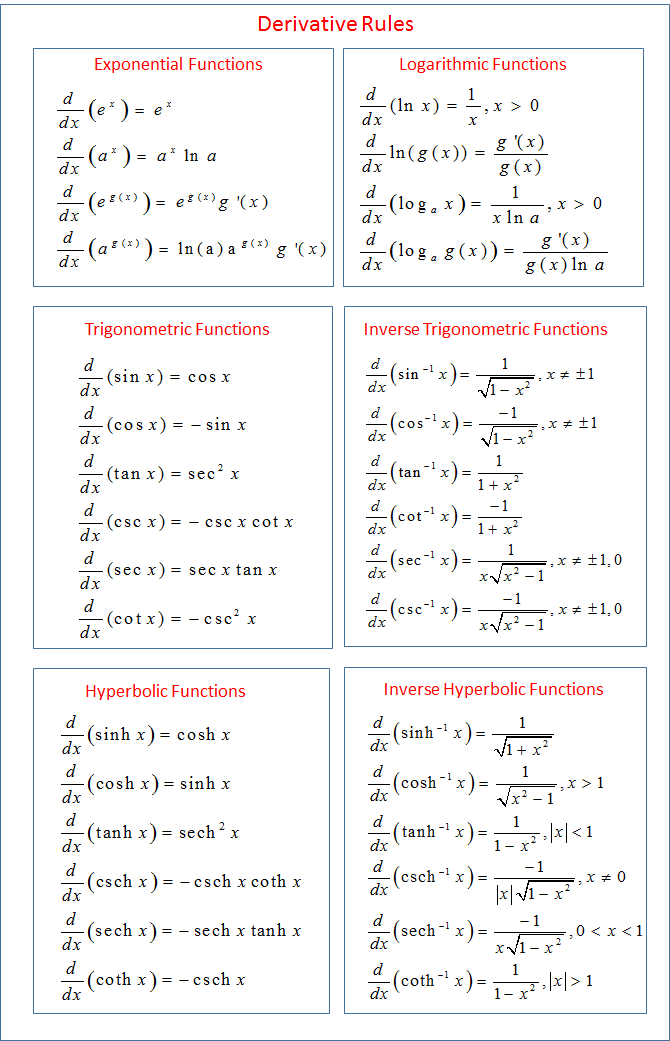
Calculus Derivative Rules (video lessons, examples, solutions)

Beautiful Work Differentiation Formulas For Class 12 Chemical Reactions
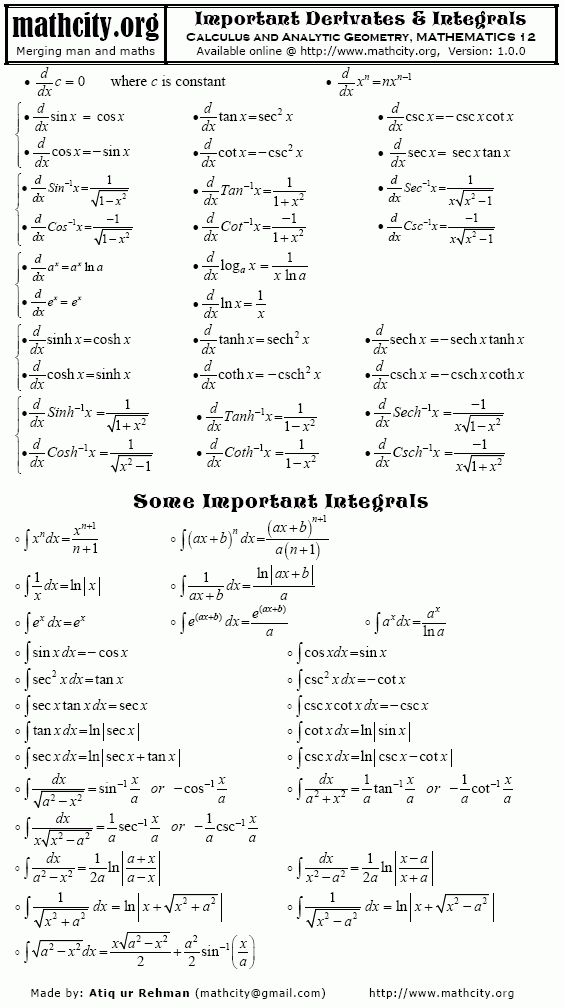
Important Derivatives & Integrals
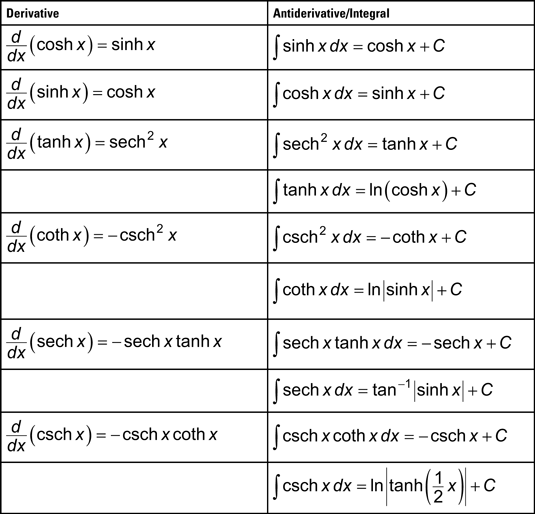
The Hyperbolic Functions in a Calculus Course dummies

Derivative and Integral Formula Wallpaper 2 by SawyerTHEBEST on DeviantArt
Mathematics Derivatives and Integrals Chart PDF
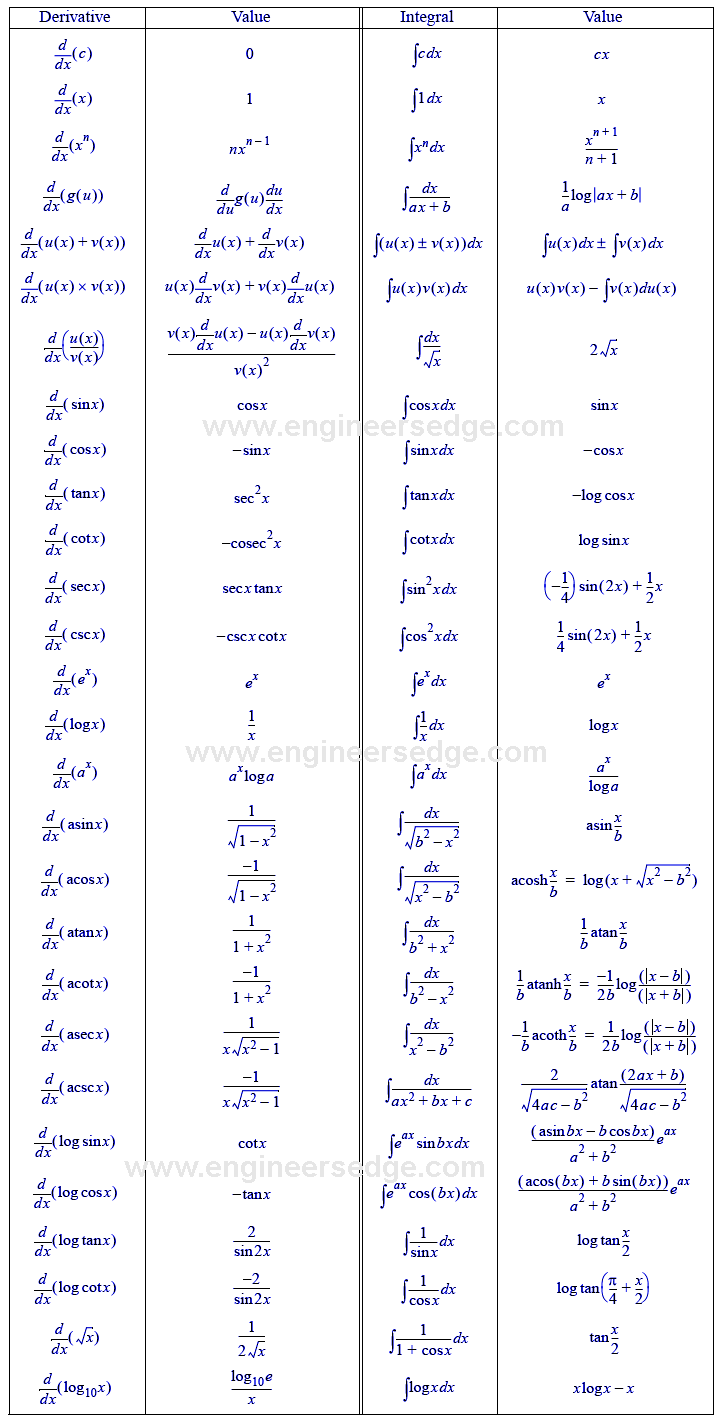
Derivatives And Integrals Formula Sheet Management And Leadership
3.7 Derivatives Of Inverse Functions;
1, N Is Any Number.
Web Table Of Basic Integrals.
Sum Difference Rule \Left (F\Pm G\Right)^'=F^'\Pm G^' Constant Out \Left (A\Cdot F\Right)^'=A\Cdot F^' Product Rule (F\Cdot G)^'=F^'\Cdot G+F\Cdot G^'
Related Post:
