Anti Derivative Chart
Anti Derivative Chart - Sometimes, it may be possible to use one of these standard forms directly. Web we answer the first part of this question by defining antiderivatives. Here we examine one specific. 4.10.2 explain the terms and notation used for an indefinite integral. Web to identify a particular antiderivative of \(f\), we must be provided a single value of the antiderivative \(f\) (this value is often called an initial condition). To obtain the most general antiderivative from the particular ones in the table, just add a constant c. Substituting the value of 2 for \(x\) in \(f(x) = \dfrac{1}{3} x^3 + c\), we find that 4.10.3 state the power rule for integrals. Web f + g is an antiderivative of f + g on i. Web if \(f\) is an antiderivative of \(f\), we say that \(f(x)+c\) is the most general antiderivative of \(f\) and write \[\int f(x)dx=f(x)+c.\] the symbol \(\int \) is called an integral sign, and \(\int f(x)dx\) is called the indefinite integral of \(f\). What do those antiderivatives all have in common? Find the general antiderivative of a given function. Web if \(f\) is an antiderivative of \(f\), we say that \(f(x)+c\) is the most general antiderivative of \(f\) and write \[\int f(x)dx=f(x)+c.\] the symbol \(\int \) is called an integral sign, and \(\int f(x)dx\) is called the indefinite integral of \(f\). The need. Web those would be derivatives, definite integrals, and antiderivatives (now also called indefinite integrals). This can be stated symbolically as f' = f. Complicated functions can be computed from these using techniques like. These are the antiderivative formulas you should memorize for math 3b. Given the graph of a function’s derivative, how can we construct a completely accurate graph of. The need for antiderivatives arises in many situations, and we look at various examples throughout the remainder of the text. State the power rule for integrals. The antiderivative of a function ƒ is a function whose derivative is ƒ. In the present example, suppose that condition is \(f(2) = 3\); The antiderivative of a function \(f\) is a function with. Find the general antiderivative of a given function. 1 a+1 xa+1 a6= 1 logjxj a= 1 x logx ( +1+1) 1x+1 logx ( +1) 2x exponents e xe ax (loga) 1ax xex (x 1)ex e xe trigonometric functions cosx sinx sinx cosx tanx logjcosxj cotx logjsinxj sec2 x tanx csc2 x cotx hyperbolic functions coshx sinhx sinhx coshx tanhx logcoshx.. Web the fundamental theorem of calculus connects differential and integral calculus by showing that the definite integral of a function can be found using its antiderivative. This can be stated symbolically as f' = f. These are the antiderivative formulas you should memorize for math 3b. Web the table below shows you how to differentiate and integrate 18 of the. Sometimes, it may be possible to use one of these standard forms directly. We are integrating, we need to be able to recognise standard forms. On other occasions, some manipulation will be needed first. The need for antiderivatives arises in many situations, and we look at various examples throughout the remainder of the text. Find the general antiderivative of a. Here we examine one specific. The antiderivative of a function ƒ is a function whose derivative is ƒ. Explain the terms and notation used for an indefinite integral. Web we answer the first part of this question by defining antiderivatives. Parts, partial fractions, trig substitution, etc. Web those would be derivatives, definite integrals, and antiderivatives (now also called indefinite integrals). Sometimes, it may be possible to use one of these standard forms directly. This can be stated symbolically as f' = f. Web explore math with our beautiful, free online graphing calculator. Substituting the value of 2 for \(x\) in \(f(x) = \dfrac{1}{3} x^3 + c\),. The antiderivative of a function ƒ is a function whose derivative is ƒ. This can be stated symbolically as f' = f. Type in any integral to get the solution, steps and graph. For a longer list of antiderivative formulas, see your textbook. The need for antiderivatives arises in many situations, and we look at various examples throughout the remainder. Web 5.1 constructing accurate graphs of antiderivatives. To obtain the most general antiderivative from the particular ones in the table, just add a constant c. When you learn about the fundamental theorem of calculus, you will learn that the antiderivative has a very, very important property. Complicated functions can be computed from these using techniques like. Web the table below. To obtain the most general antiderivative from the particular ones in the table, just add a constant c. Web 5.1 constructing accurate graphs of antiderivatives. Complicated functions can be computed from these using techniques like. Sometimes, it may be possible to use one of these standard forms directly. In the present example, suppose that condition is \(f(2) = 3\); Graph functions, plot points, visualize algebraic equations, add sliders, animate graphs, and more. Web if \(f\) is an antiderivative of \(f\), we say that \(f(x)+c\) is the most general antiderivative of \(f\) and write \[\int f(x)dx=f(x)+c.\] the symbol \(\int \) is called an integral sign, and \(\int f(x)dx\) is called the indefinite integral of \(f\). 1 a+1 xa+1 a6= 1 logjxj a= 1 x logx ( +1+1) 1x+1 logx ( +1) 2x exponents e xe ax (loga) 1ax xex (x 1)ex e xe trigonometric functions cosx sinx sinx cosx tanx logjcosxj cotx logjsinxj sec2 x tanx csc2 x cotx hyperbolic functions coshx sinhx sinhx coshx tanhx logcoshx. Explain the terms and notation used for an indefinite integral. Web to identify a particular antiderivative of \(f\), we must be provided a single value of the antiderivative \(f\) (this value is often called an initial condition). We use the notation ∫ f (x)dx ∫ f ( x) d x to denote the indefinite integral of f f. Web the most general antiderivative of f (x) f ( x) is the indefinite integral of f f; Parts, partial fractions, trig substitution, etc. Web the fundamental theorem of calculus connects differential and integral calculus by showing that the definite integral of a function can be found using its antiderivative. What do those antiderivatives all have in common? State the power rule for integrals.
Antiderivative Rules Cheat Sheet
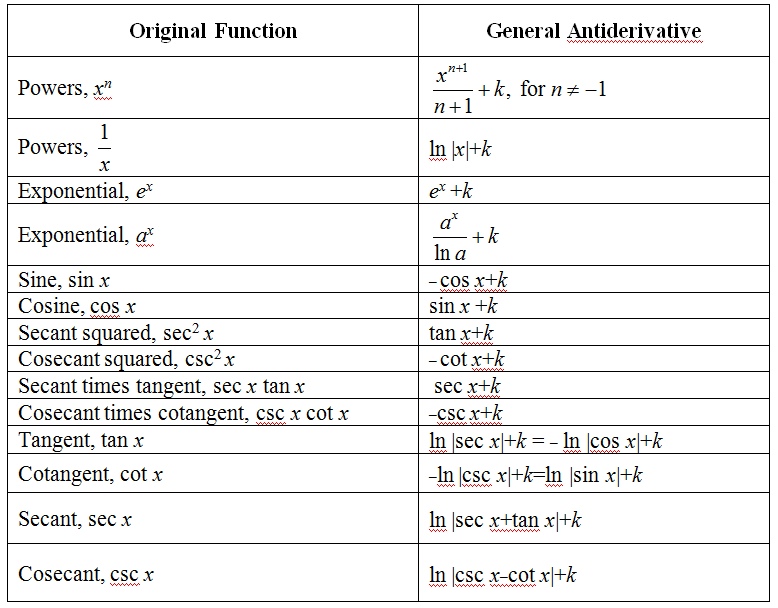
Find Antiderivative Online Selection, Save 56 jlcatj.gob.mx

6.8 Finding Antiderivatives and Indefinite Integrals (Part 5
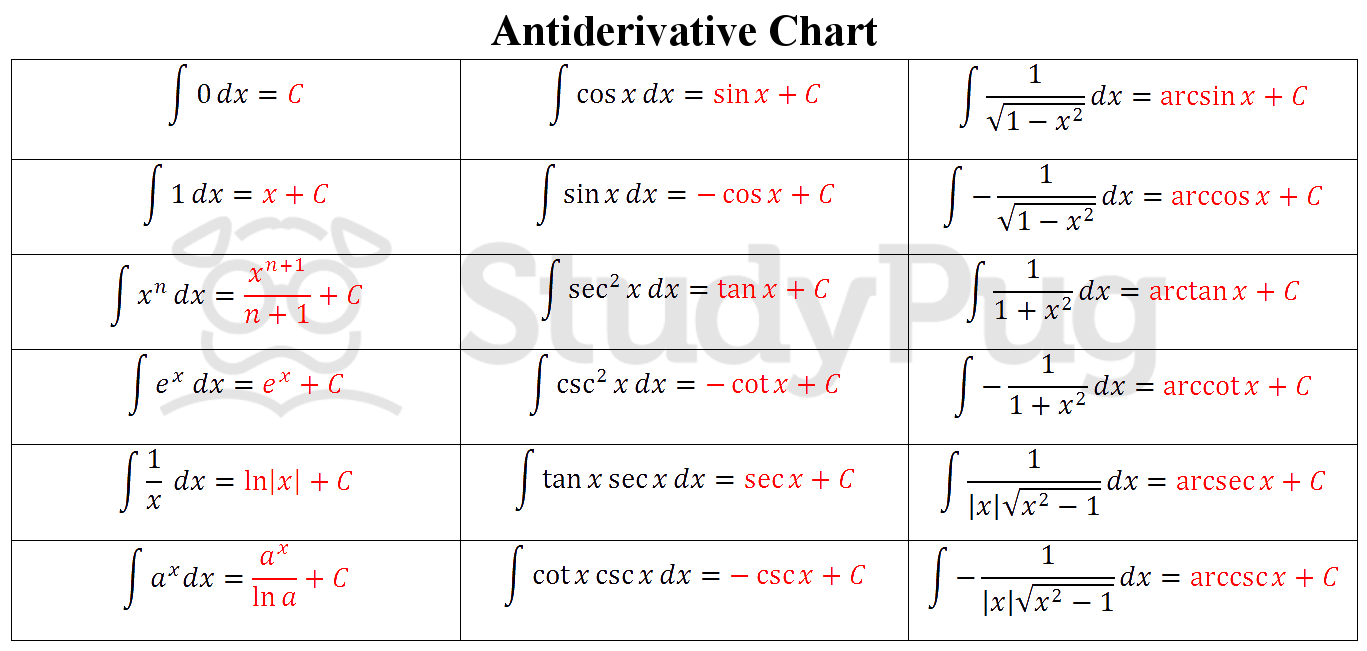
Introduction to antiderivatives StudyPug
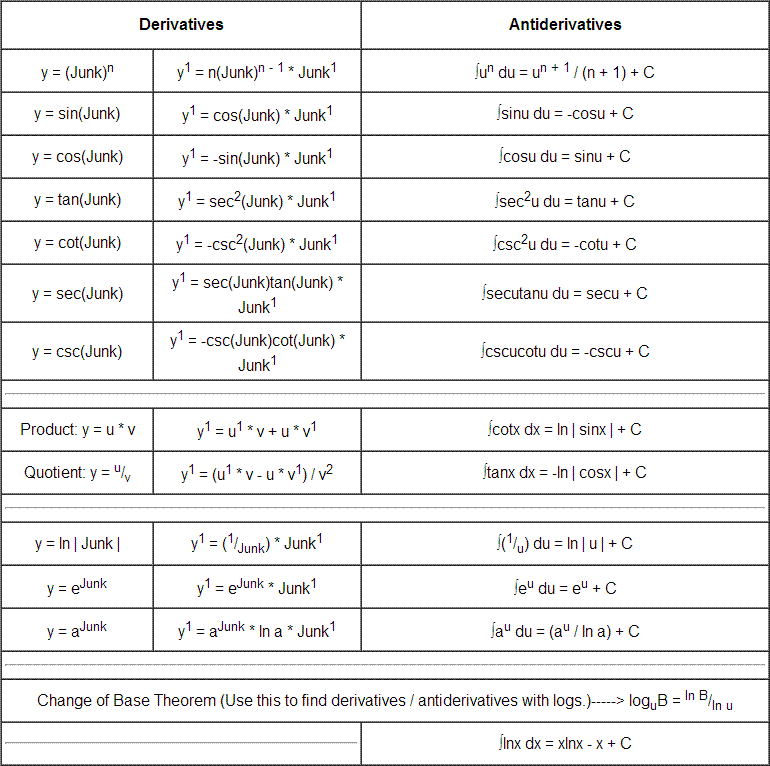
Table of Derivatives/ Antiderivatives
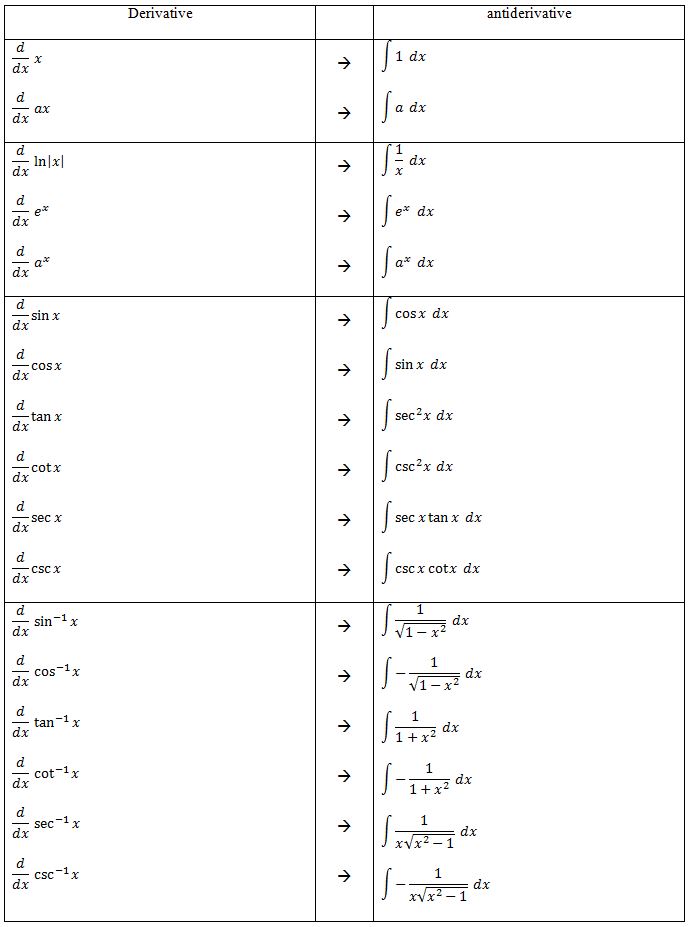
Introduction to antiderivatives StudyPug
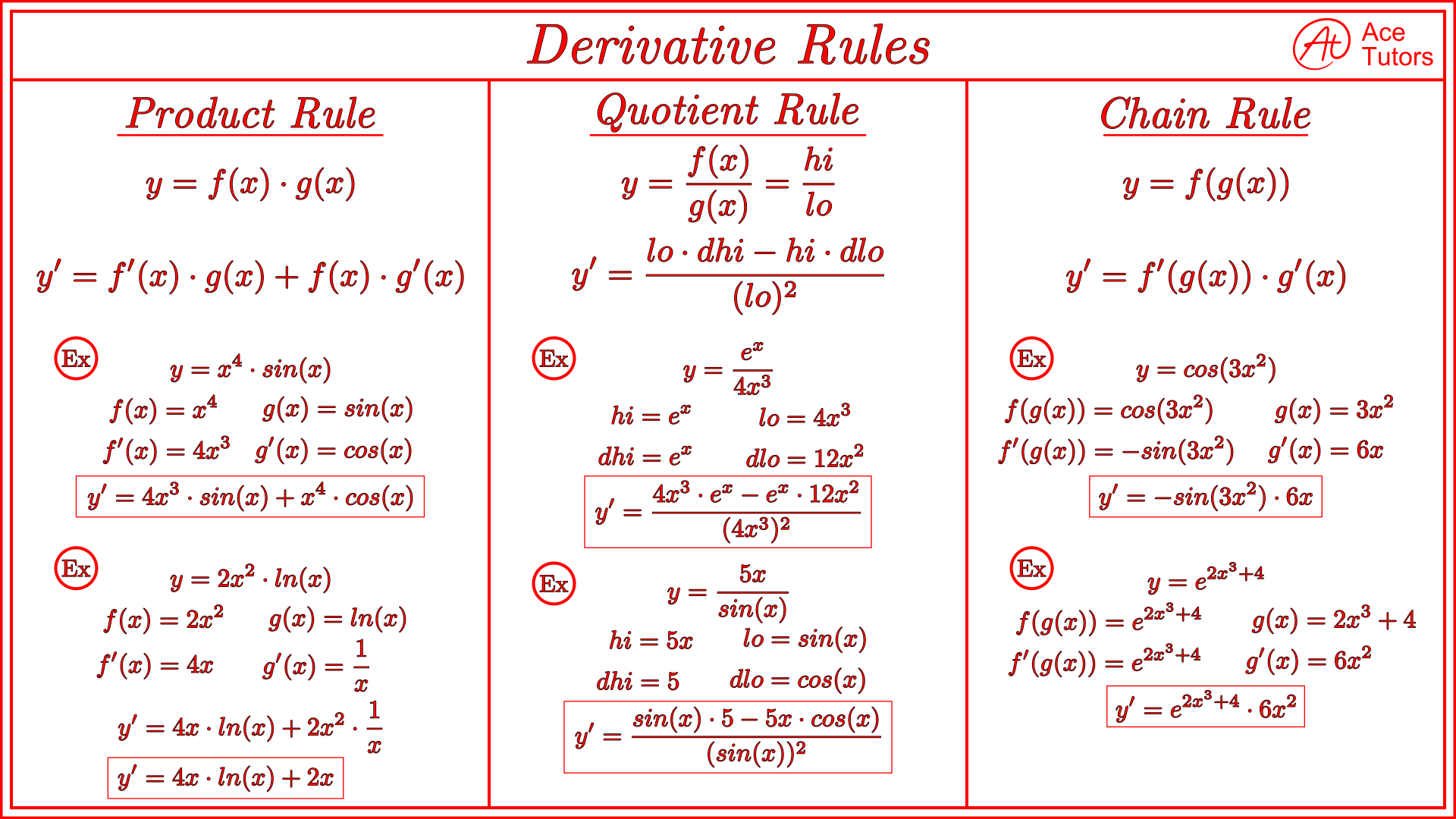
Page Of Some Important Rules Of Differential Integral Calculus Hot
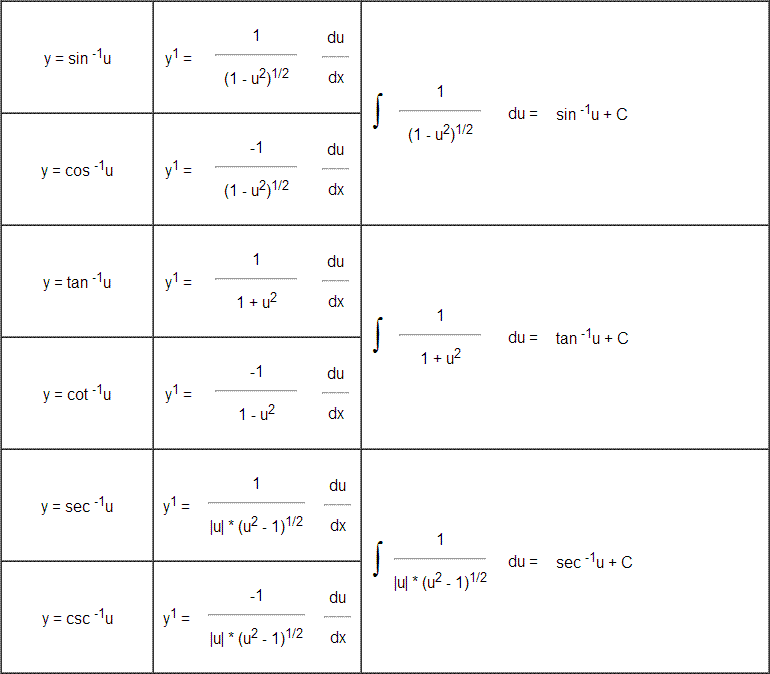
Table of Derivatives/ Antiderivatives
Antiderivative Rules

Antiderivative Graph Complete Explanation and Examples The Story of
Web 4.10.1 Find The General Antiderivative Of A Given Function.
The Need For Antiderivatives Arises In Many Situations, And We Look At Various Examples Throughout The Remainder Of The Text.
Here We Examine One Specific.
Type In Any Integral To Get The Solution, Steps And Graph.
Related Post: